Workers in some countries are a lot more productive than workers in other countries. One of the main differences is that people in more productive countries have more education. When we hear that education in a country is going up, we normally take this as a sign that productivity is rising. Question: If the signaling model of education is right, does this mean that rising education is not a sign of progress? Does it mean that rising education is actually a sign of decay?!
No and no. Holding policy constant, the signaling model of education specifically implies that rising education is a symptom – though not a cause – of rising productivity. I’ve long taken this for granted, but yesterday I created a simple model to prove it. Alex Tabarrok and Garett Jones have checked my math, but any errors are my own.
The model:
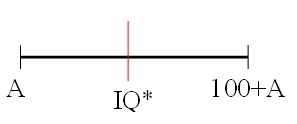
1. IQ ~ U[A, 100+A], with A ≥0.
In English, intelligence is uniformly distributed between A and 100+A, like so:
The higher A, the higher the average IQ of the workforce. We’ll define IQ* momentarily.
2. Worker productivity is proportional to IQ. For convenience, in fact, productivity equals IQ.
3. The only signal of IQ is a diploma, and a worker can only get one diploma.
4. Due to competition and imperfect information, employers pay a wage equal to the average productivity for people with their education level. WN=wage of workers without a diploma. WD=wage of workers with a diploma.
5. Each worker maximizes U=W – K/IQ, where W is the worker’s wage, K is a constant, and IQ is the worker’s IQ. K/IQ is a signaling cost; the smarter you are, the less painful you find it to get your diploma. (A≥0 because this signaling cost function wouldn’t make sense with negative A).
Given the set-up, there’s clearly going to be an IQ level such that everyone above it gets a diploma and everyone below it doesn’t. Let’s call that IQ level IQ* and solve for it. The formula for a midpoint of a line implies that for the person with IQ*:
WN=(A+IQ*)/2
WD=(IQ*+100+A)/2
The person with IQ* is indifferent about getting a diploma when:
(A+IQ*)/2=(IQ*+100+A)/2 – K/IQ*
Subtracting (A+IQ*)/2 from both sides and simplifying leaves:
IQ*=K/50
In equilibrium, everyone with IQ greater than K/50 gets a diploma. Notice that A, the ability parameter, drops out; IQ*=K/50 for all A. If A equals 0, and K=2500, then IQ is uniformly distributed between 0 and 100 and IQ*=50, so 50% of the population gets a degree. If A=40, then IQ is uniformly distributed between 40 and 140 and IQ*=50 (still!), so 90% of the population gets a degree. If you could increase IQ by sprinkling pixie dust on the population, education levels would rise despite the fact that education has zero effect on productivity.
Is it possible that the social costs of signaling are so great that more social ability leads to lower social utility? At least in this model, no. The deadweight loss of signaling is just the area under the signaling cost function K/IQ for everyone who chooses to signal:
This integral equals K*ln IQ. Plugging in the lower and upper limits yields a deadweight cost equal to:
K[ln(100+A)-ln(K/50)]
Total Social Welfare is just the population (=100) times the average wage (=average IQ = 50+A) minus the deadweight cost of signaling:
SW=100*(50+A) – K[ln(100+A)-ln(K/50)]
To verify that higher IQ increases SW, differentiate SW with respect to A:
dSW/dA=100 – K/(100+A)
Is this always greater than 0? Yes. In any interior solution, the diploma cutoff IQ* is less than or equal to the maximum IQ: K/50 ≤100+A. So K/(100+A)≤50, implying that 100 – K/(100+A)≥50 for all A≥0. Higher IQ unambiguously raises Social Welfare for interior solutions.
The same holds for corner solutions. If the cost of signaling is so high that no one gets a diploma (K/50>100+A), there’s zero deadweight cost of signaling, and raising A automatically raises Social Welfare by 100 ΔA (the population*the change in A). If the cost of signaling is so low that everyone gets a diploma (K/50<A), it’s impossible for the deadweight cost of signaling to increase any further. Raising A once again raises Social Welfare by 100ΔA.
Bottom line: When IQ rises, total signaling costs go up, but the net effect of more productivity plus more signaling is still positive. More diplomas, like bigger wedding rings, are indeed a symptom of progress. But only a symptom. Insofar as the signaling model of education (or the signaling model of wedding rings!) is true, policy analysts have to be careful not to conflate a mere symptom of progress with progress itself.
P.S. If I were submitting this model to a journal, I’d spend a lot more time checking my math. If you do find a mistake, please share it with no more than moderate scorn. 🙂
P.P.S. It wouldn’t be too hard to show that this model also implies that increasing educational equality is a genuine symptom – but not a cause – of increasing labor market equality.

READER COMMENTS
Arnold Kling
Apr 12 2012 at 1:05pm
This reminds me of embodied technical change. The company wants the better technology (higher IQ) but it comes only with newer capital (the degree)
Eli
Apr 12 2012 at 2:21pm
Does anyone know a good introductory text in economic mathematics / calculus / statistics? I want to learn more, but I didn’t do very well in secondary education. It was probably a cost of having been homeschooled k-12. Homeschooling did however, equip me to educate myself even when the material is very dry or rigorous (Saxon math series I took in high school is known for that).
Vipul Naik
Apr 12 2012 at 2:51pm
This post is good signaling of your mathematical econ skills.
Eli
Apr 12 2012 at 3:41pm
The math seems right, but I don’t understand why you would assume that K is constant. It should be an increasing function of A, no? My intuition is that as ability increases, people have to do more and more costly stuff to differentiate themselves.
If K is increasing in A, then it’s not at all clear that as A rises more people will signal.
Collin
Apr 12 2012 at 4:04pm
Bryan,
Is there anyway to show a time lag? Is it education today produces better things tomorrow? The US has the most productive economy the last 150 years but also the most education (Matt point) and multi-cultural (your point.)
1) From 1930 – 1940, it probably did not make sense on paper to high school educate women who were expected to be mothers. However during WWII, Rosie made a big difference.
2) The Greatest generation well educated the Boomers who turned the US economy from industrial based in ~1970 to the information age in ~1990.
3) Lastly, don’t college educated people generally divorce less? (Could be an age factor as well) We know you like that one.
To me, cutting back education is like the Big Pharma continuously cutting back R&D for new drugs. It supports your current profits but long run limits the growth of the company. Notice how drug prices are falling.
CR
Emily
Apr 12 2012 at 6:46pm
In this model, as you approach the point where everyone has a diploma, the gain from having all employers pay everyone the same amount regardless of whether they have a diploma increases.
M.R. Orlowski
Apr 12 2012 at 11:41pm
Eli,
I think that Khan Academy has some pretty decent calculus videos and the model that Bryan has built isn’t all too complicated, mathematically speaking, so you can learn the math behind it by watching the Khan Academy lectures up to indefinite and definite integration and perhaps you can find some online material for a more sophisticated understanding of integration.
Silas Barta
Apr 13 2012 at 3:41am
Haven’t traced through the math, but I think you mean IQ is uniformly distributed between 100+A and 100-A, not 100+A and A. The latter case would have to be something bizarre like 10 to 110 or 50 to 150.
Bryan Caplan
Apr 13 2012 at 9:44am
Silas, if you make IQ U~[100-A, 100+A], then increasing A makes the math ugly, because you have to divide everything by [100+2A] to keep the population constant. Just think about IQ as a test with a mean score of 50 on a typical population rather than the usual 100.
R Richard Schweitzer
Apr 13 2012 at 11:01am
Does this kind of modelling or “metrics” display a method of examining whether there is an increase in the distribution of “intelligence” over population groupings as the result of “increased education;” or, does it merely reinforce the assumed measures of intelligence?
Does intelligence (and its “improvements” or distributions) involve the capacities to validate or determine flaws in perceived information?
Does intelligence require the indentification and recognition of connections between separate bits of perceived information; to the extent that they have significanace or meaning to the observor; thereby becoming knowledge?
What “measures” of the effects of increased “education” deal with either of those two preceding questions?
John T. Gregg, MD
Apr 13 2012 at 1:43pm
Eli
Simon and Blume – Mathematics for Economists
ISBN-13: 978-0393957334
http://www.amazon.com/Mathematics-Economists-Carl-P-Simon/dp/0393957330/ref=sr_1_5?s=books&ie=UTF8&qid=1334338672&sr=1-5
Rich Seiter
Apr 15 2012 at 12:30pm
Assuming intelligence is a uniform distribution seems very questionable (IQ is generally considered to be close to a Gaussian). To my mind this calls the whole analysis into question. Am I the only one bothered by this? Can anyone offer a justification why the uniform assumption is OK?
David Jinkins
Apr 15 2012 at 1:13pm
Mathematical quibble:
In 5. above you say “Each worker maximizes U=W – K/IQ” but there is nothing to maximize there. What you mean is that each worker chooses the maximum of {W_D – K/IQ,W_N}. Since there is a continuum of workers, each individual worker can forget about his effect on aggregate wages.
Substantive quibble:
You need to carefully define your equilibrium concept, as signaling models usually have multiple equilibria. Firms paying an average wage is not a primitive, and you really should say something about firm beliefs (this is a signaling model, after all!). Suppose that each firm hires one worker, and has a linear production technology in the IQ of the worker (I think that is what you are getting at above). As above, there is perfect competition, and firms can only observe whether or not a worker has a diploma.
An equilibrium is firm beliefs over the IQ distribution of diploma and non-diploma holders and wages such that:
1. given the wages, optimal worker choices are consistent with the IQ distributions believed by the firm.
2. Given the IQ distributions, it is optimal for firms to offer the wages (zero profit).
In this case there is an equilibrium for any K in which firms believe that non-diploma holder distribution is U[A,100+A], and the diploma holder distribution is a mass point at A. Given that belief, it is optimal for no one to get a diploma (1). Given that no one gets a diploma, the firms beliefs are consistent (2).
I also didn’t carefully check my logic, so let me know if I am missing something.
Comments are closed.